Strains in 2D#
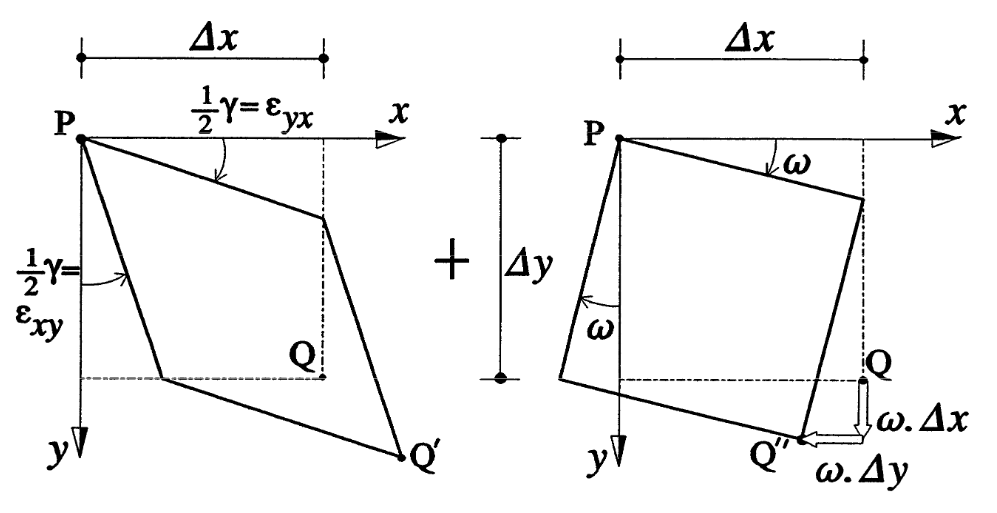
Strains in 2D can be represented in a matrix (specifically a second-order tensor) as follows:
\[\begin{split}\begin{bmatrix}
\Delta u_x \\
\Delta u_y
\end{bmatrix}=
\begin{bmatrix}
\epsilon_{xx} & \epsilon_{xy} \\
\epsilon_{yx} & \epsilon_{yy}
\end{bmatrix}\begin{bmatrix}
\Delta x \\
\Delta y
\end{bmatrix}+
\begin{bmatrix}
0 & -\omega \\
\omega & 0
\end{bmatrix}\begin{bmatrix}
\Delta x \\
\Delta y
\end{bmatrix}\end{split}\]
The first part is a deformation, the second part is a rigid body rotation.
This is treated in chapter 1.2 of the lecture notes Introduction to Continuum Mechanics (Hartsuijker and Welleman, 2007).
