Shear stresses

Shear stresses#

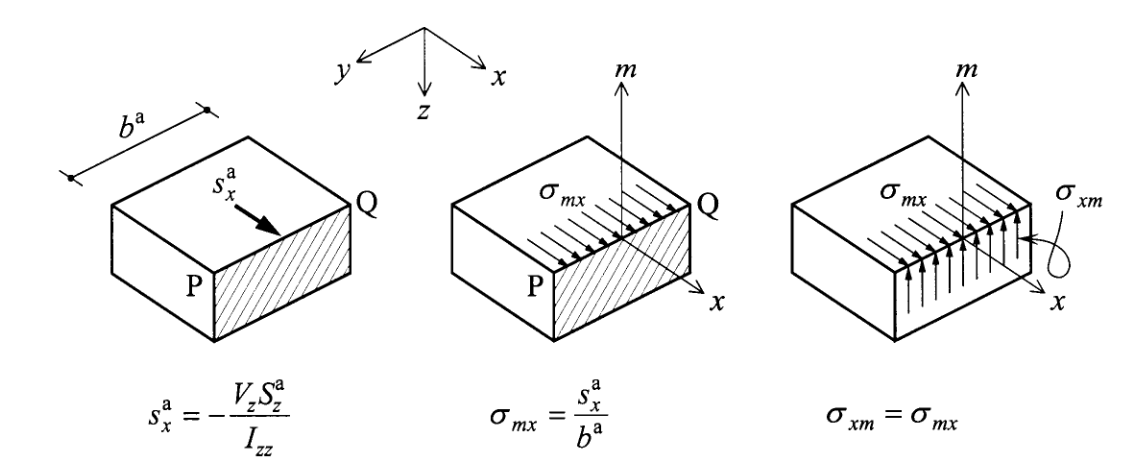
../../_images/image_shear.png

Fig. 46 Figure 5.13b,c,d Hartsuijker and Welleman (2007)#

Longitudinal shear stresses#

The shear force per unit length in the longitudinal direction is given by:

\[s_x^a = -\cfrac{dM_z}{dx} \cfrac{S_z^a}{I_{zz}} = -\cfrac{V_zS_z^a}{I_{zz}}\]

or alternatively:

\[s_x^a = - {V_z}\cfrac{{{N^a}\left( {{\text{due to }}M_z^*} \right)}}{{M_z^*}}\]

The shear stress is given by:

\[\sigma_{mx} = \frac{s_x^a}{b^a}\]

This is treated in chapter 5.1 and 5.2 of the book Engineering Mechanics Volume 2 (Hartsuijker and Welleman, 2007).

Cross-sectional shear stresses#

The shear stress in the cross-sectional direction is givenby:

\[\sigma_{xm} = \frac{s_x^a}{b^a}\]

This is treated in chapter 5.3 and 5.4 of the book Engineering Mechanics Volume 2 (Hartsuijker and Welleman, 2007).

Exercises#

Contents